Okay, I want this post to be as positive as possible. But to do that, I’m going to have to feel sorry for myself for a little bit. Feel free to ignore the next few paragraphs.
I’m currently in bed, where I’ve been for most of the day. I’ve got some sort of cold/fluey type thing that I’ve been trying to ignore for the last couple of days, but it finally got to me. The good news is that I wasn’t going to be teaching today anyway, so I didn’t need to rush to organise classes for extras, but I did miss out on the PD I was supposed to go to.
I absolutely hate taking days off, because I feel like I’m letting my students down. It’s a struggle to fit in everything I want/need to do with my classes as it is, before allowing for lessons my body refuses to let me get to. I’ve had a good run with not having to take many sick days, so I guess I can’t complain too much.
The fact this is the first week of term just makes me feel even more pathetic. I imagine there are certain critical types who would want to say how this is typical of the laziness of the teaching profession. I suggest those people visit me to say that to my face. So they can enjoy me coughing all over them as they say it.
So anyway, I am feeling a lot better than I did this morning and I’m fairly sure I’ll be back at school tomorrow.
The first two days of term were a lot better than today. Monday felt in many ways like the first day of the year rather than just the start of a new semester. Part of that was changing electives to ‘Robotics’, though all the students in that class were also in my Web Development class last semester, so it didn’t take long to do the introductory stuff.
But I’m also seeing the new term as a chance to reset with my Year Nine class, just a little bit. I’m not saying that the first half of the year was terrible, because it wasn’t, but there’s lots of things I want to work on and experiment with. I spent the start of Monday’s lesson explaining to my students a few of the changes I’m making, but also explaining that I want our focus to be less on “getting the right answer” and more on thinking as mathematicians.
This class is very competitive, which can be useful at times, but has sometimes meant their aim has been at completing the work before others (or for the weaker students, just giving up when they can’t keep up). I’m trying to get them to aim for improving their own ability in thinking mathematically, even if that means working slower at times.
The class was very disappointed when I told them New Things Thursday was no more. When I explained that New Things was moving to Monday, they were both happy and annoyed that I’d tricked them (and fair enough, but I couldn’t help myself).
New day needs a new graphic!
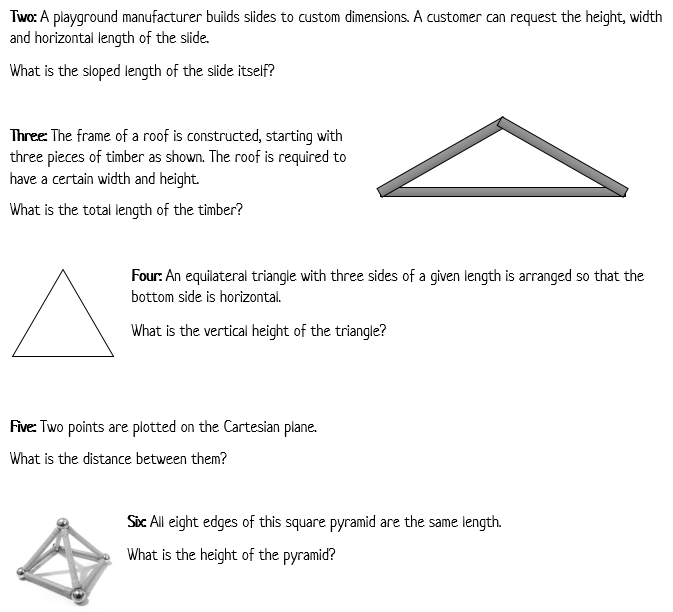
This week, New Things focussed on new problem solving techniques, rather than on new content. In the past, I don’t think I’ve spent enough time teaching problem solving explicitly. The difficulty with teaching this is, though, is avoiding listing a specific set of steps to follow – I want my students to think about the problem mathematically, not follow a recipe.
I gave the class this problem:
A flagpole is secured by attaching a fixed length of wire between the top of the pole and the ground.
How far from the base of the pole is the wire attached to the ground?
The students immediately complained that they couldn’t answer the question. “There’s no numbers there!” When I asked them to clarify, they said the lengths of the wire and the pole were missing.
The point I was trying to make was this: You don’t need ‘numbers’ in order to think about a problem mathematically. So with encouragement from me, the class set about exploring the problem:
- We drew diagrams of the scenario.
- We defined the variables involved and gave them pronumerals.
- We wrote equations using those variables and Pythagoras’ Theorem.
“We still don’t know the measurements!” came the complaints. So I suggested they each write down reasonable measurements of the pole and the wire, and make an estimate of our unknown distance. Then I set these two tasks:
- Using your own measurements, calculate the distance from the base of the pole to the wire attachment.
- Make the same calculation using your neighbour’s measurements.
A few observations I made while students were doing this:
- Some struggled to choose an estimate, including a few who usually excel in this class. This is a type of thinking they aren’t yet comfortable with, which was exactly what I was looking for.
- Students were checking their answers against their estimates, and asking me if they thought their answers didn’t make sense. (Yeah!)
- Conversations naturally developed around the room, with students comparing and discussing their answers with each other. (Double yeah!)
- Students were sometimes puzzled by their results. Some asked me questions like, “If I add a meter to the ground, why doesn’t that add a meter to the wire?” Then, with a little prompting, they were able to reason through their confusion using both the equation and their description of the scenario. (Yeah! Yeah! YEAH!)
Suffice to say, I was pretty thrilled with how it went.
There’s still plenty of improvements I want to make to this style of lesson. For example, I wish I had a photo, or even a 3D model to go along with the scenario. I wish I could get the class to ask the question (or other related questions) without me writing it out explicitly. Also, the fact this was part of our unit on Pythagoras’ Theorem made it fairly obvious that it would be part of the solution. But I think this was a good start to getting my students to think about problems differently.
I gave the students a handout with problems of progressive difficulty (links below) to tackle for the rest of the lesson. I gave them the freedom to choose which ones they wanted to do, by cutting and pasting the questions into their books. Some of them still aren’t thrilled about the cutting and pasting thing – they still prefered to rewrite the question themselves. Oh well, I guess some 15 year olds are just too cool for glue. I’ll win them over yet.
Downloads: