I think it’s a tough balancing act to find the point where students can investigate mathematical ideas independently. Not enough guidance, and the class will stare at me, wondering what they’re supposed to even be doing. Too much help, and they just start guessing at the answer that will get me to move on from this prompt. The sweet spot is when they learn a new idea that they’ve discovered themselves, which is “coincidently” the content I was trying to teach all along.
Today I wanted to give my year nine students the chance to “be mathematicians”: to pose a conjecture, then either prove or disprove it. We’ve been working on expanding, and we’re at the point of discussing expanding binomial products and preparing to introduce factorising. I thought letting students discover that
(x + a)(x + b) = x2 + (a+b)•x + ab
was a good opportunity to prove a conjecture, as well as practice expanding.
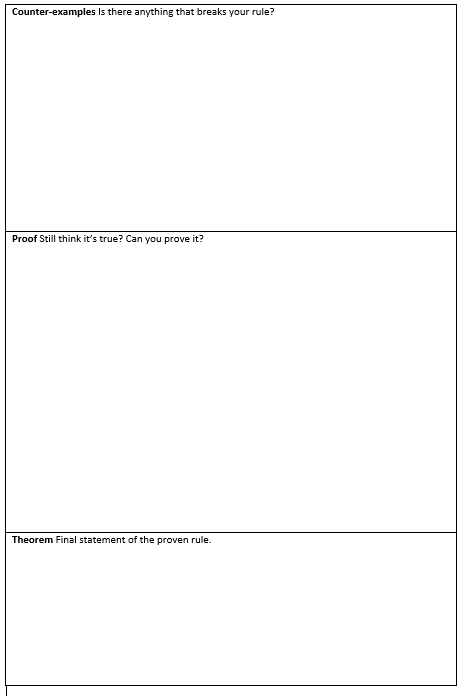
I produced the following template. You’ll notice that there’s nothing about expanding in particular in it, so hopefully I’ll be able to reuse this in other lessons.
Download: conjecture proof theorem template.docx
I broke the students into groups of four. They each had to create their own example of a binomial product, then they had to expand the examples of each person in their group. As they did this, I wrote some of their answers on the whiteboard.
From there, they had to see if they could notice any pattern, which became their conjecture. Some needed a little prompting, but someone in most groups noticed, leading to discussions within the groups, about whether it really did fit the examples. Hooray!
Next, they had to see if they could find any counter-examples. Of course, the rule that we were leading to is true, but they didn’t know that yet. Also there was still a bit of refining to do to the conjectures, as most hadn’t taken non-monic cases into account (though not in those words). Luckily, one student in the class had used (2x + 4)(x + 3) as their original example, and as it was on the whiteboard many students had noticed it. To some students, I suggested they explore this further: could they find any other examples that didn’t work?
Next step was the proof. Most needed a little help at the start – the idea of substituting the numbers with pronumerals was a step of abstraction they weren’t quite ready for yet. But they did pretty well from that point on.
A couple of students finished early, so I managed to snap pictures of their work. They were in the same group, so their examples are the same:


(Not sure why the second one is backwards.)
By the end of the lesson, I was even able to get some students started on the same process, but this time with perfect squares.
Most of the class took to this activity really well. Some did not – I think they struggle a little with staying focussed when tasks are slightly more open-ended (not that this task was particularly open-ended, but the kids didn’t know that). Is this the sort of thing they’ll get better at with practice, or do I need to make the activity more structured for them? As I said at the start of this post, that balance can be difficult.
But it was still fantastic to get the class thinking through the properties of expansions themselves. And not a “FOIL” in sight!