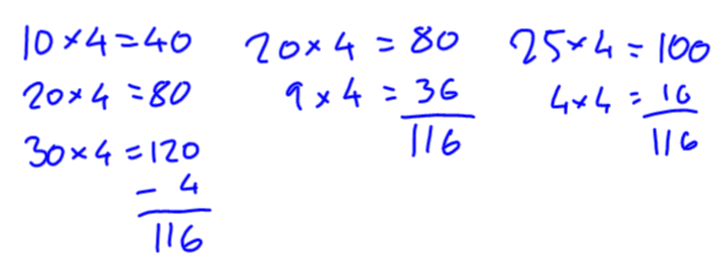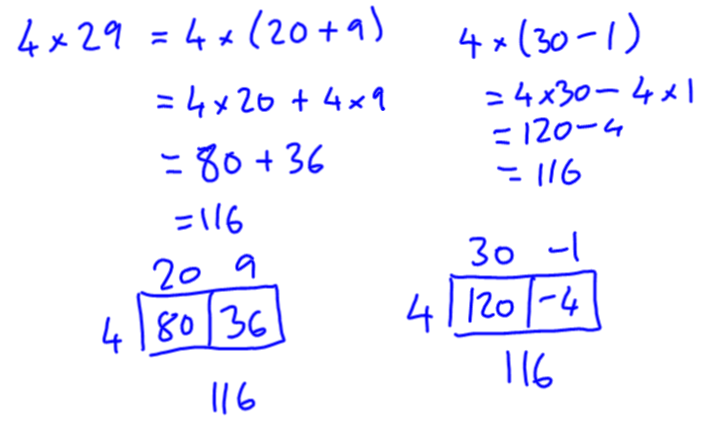Teaching the distributive law is an interesting prospect. Because really, if students know how to multiply numbers with more than one digit they already use the distributive law, even if they don’t know it. But if you write this on a board:
a•(b + c) = a•b + a•c
and expect kids to understand it, you’ll be met with blank looks. The challenge is not in knowing the law, but in connecting it to prior understanding of numerical multiplication and extending that understanding to algebra.
When Year 9 and I started our unit on Expanding and Factorising (which admittedly was weeks ago, we’re nearly finished the next unit now), I posed a seemingly simple question: what is 29 × 4? The class worked in groups, and I enforced a couple of rules: they couldn’t use calculators and they couldn’t use the standard written algorithm.
Looking back now, I really should’ve created a better graphic than just drawing on the IWB…
Most were clearly happy with themselves for finding the answer, but I ruined that by writing 116 on the board as we came back together as a class. Just giving me the answer was no longer good enough. The groups needed to explain how they got their answers.
As a side note, I find this a constant struggle with my students. How do I get them to unlearn the idea that “getting the answer” is everything, and think more in terms of understanding the problem?
Anyway, it turned out that amongst the groups there were a few different methods used.
I think most readers will know where this is going. Though the methods used were different, they were all dependent on the distributive law. I presented some of their solutions to them in a different form.
This was a good launching point for talking about expanding. After the class did a couple more numerical examples, we started introducing variables into it.
Of course, I was silly enough to do that part on the whiteboard instead of the IWB, so I don’t have an image of it 🙁
Often with algebra, we are teaching rules and patterns that students already know are true, but they are not familiar with the language and structure of algebra. Yes, expanding 2(x+3) is an abstract idea, but 29×4 is itself an abstraction, really. A useful strategy is to help students realise that algebra is often just a layer of abstraction over concepts they already know and understand.