So I need to start this post with an admission: I got this activity from our textbooks. In my own defense, I really only took the initial idea from the book, but then made up the rest of the lesson myself.
So the idea I “borrowed” today was about rugby league and using trigonometry to calculate the angles involved. In particular, trying to work out the optimal position to kick the ball from to make a conversion.
Now, quick aside for those people who know nothing about league. (Sorry to NSW and Queensland readers who are about to see me butcher this explanation. I’m from Victoria, which is an AFL state, not league.) Aim of the game is to score a “try” by crossing the ball over the goal line. If a team scores a try, they get a chance to “convert” it by kicking the ball off a kicking tee through the goals to get a few extra points.
Now here’s the bit that’s relevant to the lesson. The kicker has to place the ball in line with where try was scored, but they can move forwards or backwards wherever they want.
So the textbook basically started by asking something like “Where should the ball be placed to maximise the chance of scoring?”, then proceeded to give a whole heap of smaller questions to step the students through the task. In the process, stripping out all the interesting thinking parts of the investigation and turning it into a set of mechanical steps.
I wanted my students to develop the questions themselves, and I certainly didn’t want them to learn about rugby league by reading the boring description in the book. (Again, my Victorian students don’t really know understand rugby league at all.) So in my preparation, I searched YouTube for “rugby league conversions” and found this video. I didn’t show the entire nine minute thing, just a few of the conversion kicks.
(I also showed part of this video of dreadful conversion attempts at the end of the lesson.)
I gave the kids two minutes to talk in their groups about any questions they had after watching the video. I then asked them to share these with the class. Initially, no-one wanted to answer, but after a little bit of prompting, the floodgates opened:
Sorry for the terrible writing and English. I was trying to get these written on the board as quickly as possible!
Some of their questions were quickly answered by refering to this page showing the dimensions of the field and goals. After a bit more discussion, we set about trying to work out the best position to kick from, to maximise the goal angle to kick through.
I assigned each group a different distance from the goal post to investigate. This divided the labour really well, as within groups different students could investigate different kicking distances to collect a wider range of results.
This worked really well – around the room there were kids discussing angles and distances, drawing diagrams and setting out tables to summarise their results. Groups were discussing what they needed to do to figure out the problem. And in the midst of it all, students were using trigonometry to work out their angles.
The best thing? Beyond the initial prompt, most of this happened without my input. I did have to give help around the room at various times, but students were willing to figure it out together in their groups. They also started using trig without me telling them to!
Though in fairness, it was reasonably obvious given we’re in the middle of our trig unit…
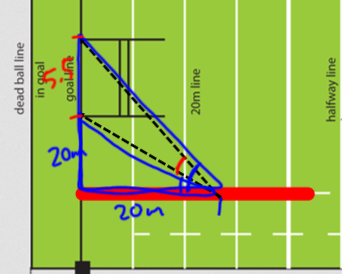
Diagram I drew on the board at one point, trying to explain… something. I’m sure it made sense at the time.
We didn’t quite get to finishing everything off, but that’s mostly because students kept posing new questions and hypotheses to investigate. A few students thought making the distance from the goal line the same as the distance of the try from the post was the best approach, and another said that 45° to the near post was best. It was great to see them slowly realise these were exactly the same thing! It was also great to see them realise their hypothesis wasn’t completely correct, and start searching for more data to figure it out.
I’m excited to see where we end up on Monday 🙂
Update: Follow up to this lesson can be found here: https://www.primefactorisation.com/blog/2014/11/26/kicking-goals-using-technology/