So I ended my last post with the words “I’m excited to see where we end up on Monday”. And now it’s been a month since I actually taught that lesson, and I haven’t blogged since then. Oops. Let’s see how I go remembering what we did…
Quick recap (though I really suggest you read that last post): we were trying to determine the optimum position to kick a rugby ball from to maximise the angle towards the goal the kicker has to aim for. Students worked in groups to figure out a method to calculate the angle, and tried various distances to see what angle resulted.
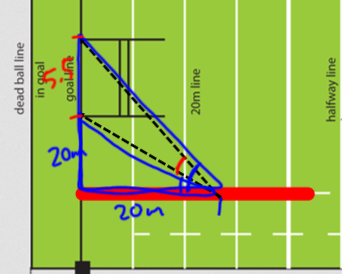
Diagram I drew on the board at one point, showing some of the angles we were calculating.
The method that each group eventually used was to calculate the angles in the two right-angled triangles, then subtracted the small one from the big one to get our kicking angle.
Students had calculated angles for a few distances by hand, but this was going to be a slow process. So, I suggested they use their computers instead to do the boring manual work. I didn’t specify what software they should use, though I did give them some hints.
The awesome thing about this was that it forced them to think about about the problem abstractly. To get the computer to do the work, they needed to specify how to get the solution generally. So, here we are in a trigonometry unit, and students are inventing and using variables themselves to help solve their problem.
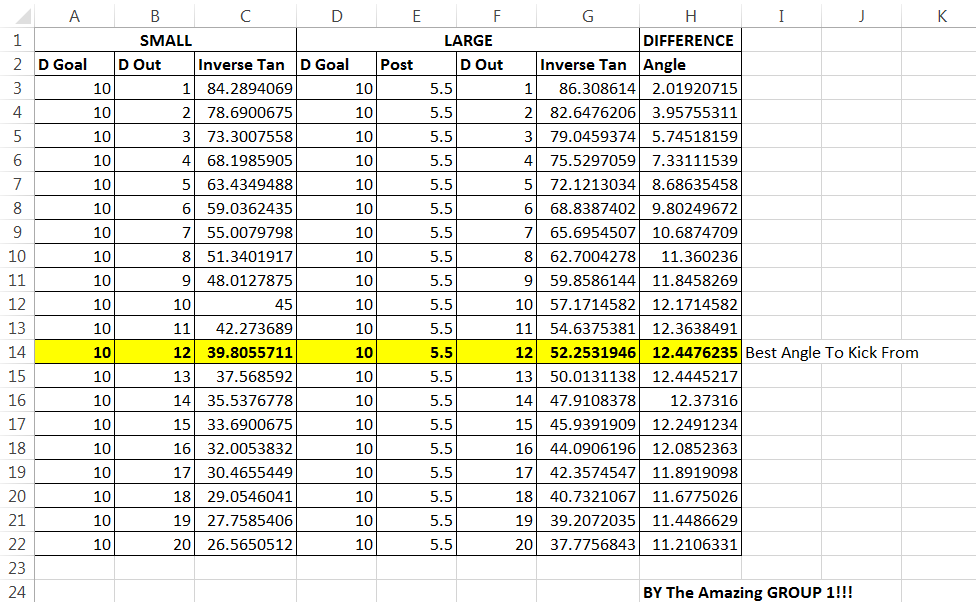
Some groups used Excel:
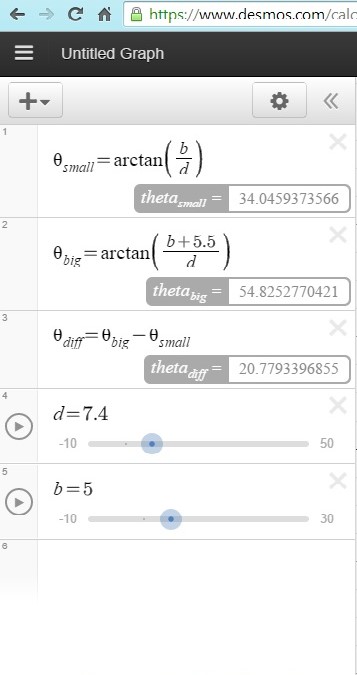
and others used Desmos:
One group was using Geogebra too, but I didn’t get an image of that.
Each group basically used trial and error, but got the various software to do the calculations for them. I loved the way this showed students the power of thinking algebraically. By using variables, they could generalise the problem. Desmos makes this use of variables explicit. But even using Excel, where there are “Cell References” instead of “Variables”, the same kind of abstract thinking is needed.
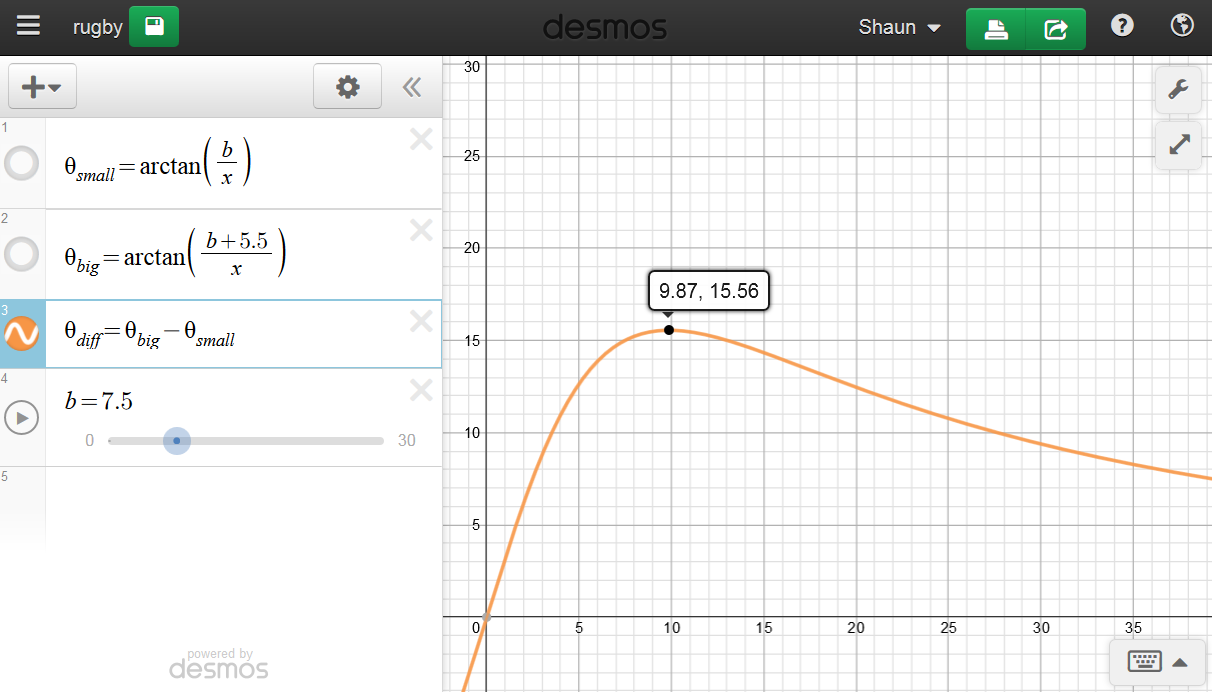
As this was a Year Nine class, we didn’t go beyond using trial and error. But afterwards I did play around in Desmos for how you might approach it in a class involving calculus:
In this case, “b” is the distance from the goalpost to where the try was scored, and “x” is the distance from the try line where the ball is kicked.
My major take-away from this lesson: the use of technology should always support getting students to think deeper about problems. Get them to think abstractly. When the boring manual work is removed by the appropriate use of technology, students are freed to experiment, to change the way they approach problems without the cost of losing a large chunk of lesson time to busy work.
What I’ve been doing lately
So, there are a few reasons why I haven’t posted anything for a month. My non-classroom responsibilities have seen me busy with VCE exams, preparing students subject choices for next year, and a few days out of school at various trainings and meetings. Also, my life outside of school suddenly got very busy as well. Don’t worry, everything’s good, but time to blog seems to be less available than it once was. But I’m hoping that as the year starts to wind up, I’ll be finding more free time to clear out my backlog of blog post ideas 🙂