This last week saw my Geometry classes finish off our introductory Reasoning and Logic unit, which means we’re ready to go with setting up the basic building blocks of geometry: the Undefined Terms.
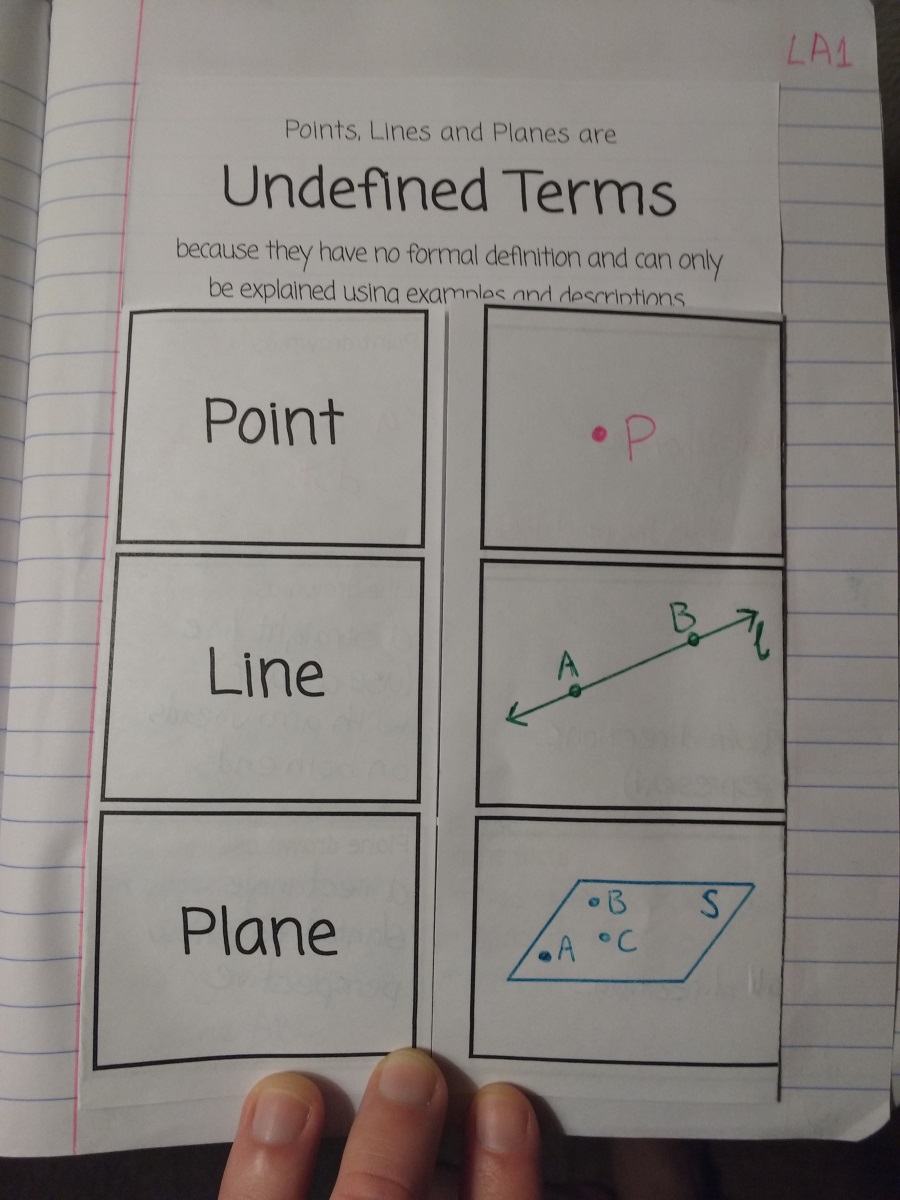
I took similar notes last year, but they were constrained to a table on half a letter sheet. This year, I redesigned it to give us a bit more space.

My students questioned why we have terms that are undefined, especially after we went over the importance of good definitions in our last unit. I reminded them that definitions use existing terms to define new terms, but that can’t happen unless we have these few terms to build everything else on top of. (Is this a good explanation? Let me know if you know of a better reason to justify the undefined terms.)
Next was our first set of postulates and definitions for the year.

A change from last year is that these postulates are glued directly onto a notebook page, rather than folded inside a foldable. This is due to a decision I’ve made this year: all postulates, theorems and definitions will be visible immediately on the page they’re on. Examples, proofs and other content can be hidden in foldables. But if I’m going to expect students to look up certain parts of their notebooks frequently, I should make those parts easy to find.
Before we wrote these down, I did a little activity to get my students thinking about why these are true. I drew two points on the dry-erase board.
I didn’t get any photos of this, so I’m re-enacting these on my computer.
Then I asked if any volunteers could draw a line through them. That went well enough:
I asked if they could do this wherever I put the points. The consensus was that they could. So far, so good.
Then I asked if anyone could draw a different line that also went through the two points. Some kids started saying yes, but then took that back once they realized they didn’t know how to do it. One even got as far as standing at the board with a marker, before handing it back to me and saying it couldn’t be done. My plan had worked as I’d hoped. The class had figured out Postulate 1 without me having to tell them what it was.
After we had written Postulate 1 down, I set my next challenge. Draw two lines that intersect at one point. No-one wanted to stand up to do this, because they thought it might be another trick question. But eventually, I coaxed one student to the board, who drew something like this:
Then I asked for two lines that didn’t intersect at all. I heard a student say the word “parallel”, so I handed her the marker:
Then I asked if anyone could draw two lines that intersected twice. But they knew my game by this point, and let me know it couldn’t be done. So it was time to write Postulate 2.
We didn’t get through the rest of the notes in this lesson, but I’ve written them up in my notebook anyway.
If you want to download these, you can do so here: