“Index Laws” is one of the topics that students at my school seem to struggle with. Okay, sure, they can use the laws, and when given an expression they can usually simplify it. But I’m convinced that they actually don’t understand indices as well as they think. There seems to be a lot of “pattern matching” going on, without much thought to what that little number above the other number means.
One lesson, I wrote 34 on the board and asked different groups of students what it meant. The first answer was always “three to the power of four”. When I asked them to explain what that meant, they confidently told me it meant “4 is the number of times you times the 3”. Which sounds right, sort of.
But when I asked them to explain what number 34 is equal to, I got some very concerning answers. Some told me it was 12. Some reasoned that 32 is 9, so this must be double that: 18. Others knew it was 3×3×3×3, but reached for the calculator to work out what that was. Surely they can do 27×3 in their heads? It wasn’t that long ago we were using the distributive law with numbers.
My class knew what indices were. But they didn’t understand them.
So what do you do when you misunderstand something about numbers? Reach for the number line!
Now, my students are pretty resistive to using number lines. They seem to think they’re too simplistic and beneath them somehow. They think because they can imagine what they look like in their heads, they don’t need to have it physically in front of them. But they usually change their mind once we do get them out, because they work. Of course, they’ll forget that and complain about them again next lesson. (Then there’s the fact they keep calling them “time lines”…)
So, multiplication is repeated addition. That’s obvious … or is it? I think that’s something we take for granted that kids know. But I wonder if years of memorising “times tables” helps kids hide that they don’t really get what “timesing” (as my students insist on calling multiplication) is.
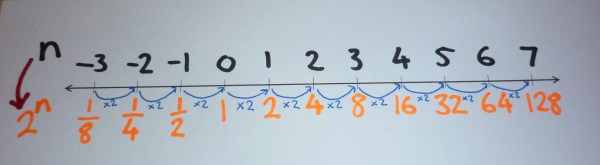
So I had students write the integers -3 to 7 along the top of a number line. I asked the class, “If we choose a value on the line, and move on space forwards, what happens to the number?” The immediate response was “you go up by 1”. I’m trying to get my students to be more precise with their language, so after discussing a little bit, this was changed to “adding 1”.
“What if we chose to add something other than 1?” I asked. So, underneath the line, we wrote numbers so that each step was adding 3. A few students noticed that the numbers along the top and bottom were related – you can just multiply by 3. Which a bit more discussion, we realised you could move between the two sets of numbers by multiplying or dividing by 3 as appropriate.
“Does this work for other numbers?” one student asked. (Hooray!) “I don’t know,” I said, “maybe we should try and find out?” (By now my students are well aware that when I say “I don’t know”, I really mean “I do know because I planned the lesson this way, but you’re not going to get me to do your thinking for you.”) So, students chose their own numbers to add.
Using 1/3 was only my idea after the lesson. I wish I had gotten at least some of my students to try it with a fraction.
So I asked another question: “What if when we make a step, we multiply instead of add?” After a discussion about needing to start at 1 instead of 0, we did a few number lines using multiplying.
If you’re the type of person who reads a blog like this, you’ll know where this is going – the result is the powers of whichever number we choose.
Once you get started with this, a lot of the rules related to indices start falling into place. Negative indices? Well, what number do you multiply by 2 to get 1? What number do you multiply by 2 to get 1/2? Very quickly, students were able to tell me that 2-10 is 1/1024, without me having to tell them that a-m = 1/am.
How about am×an = am+n? It makes much more sense as, say, “multiplying 5 four times then two more times is the same as multiplying 5 six times”.

Sorry about the bright colours. Did you know they make “neon” Sharpies? Did you know I already owned some but had forgotten? I got a bit excited when I found them.
This is where we stopped, but pushing this idea further makes this lesson just as appropriate for higher levels. What numbers fall between the integer indices? Is there a way of moving from from the bottom row back to the top. Get this: I actually had a student ask that last question. I had a year 9 student ask me about logarithms! I wonder if the confusion my Year 12s have around logs is a result of being confused about indices? In the same way that tables can hide misunderstandings of multiplication, I wonder if the textbook presentation of index laws can hide misunderstandings of indices?