The Prime Factorisation of Me

I’ve decided to make functions the key focus of the first few units of Algebra 2 this year. I mentioned this in my post about my SBG skill lists:

This year, the start of the [Algebra 2] course is going to
focus on functions, transformations and inverses. For quadratics that means only dealing with vertex form, as well as showing the relationship to square roots. We’ll cover all the functions that way, before coming back to all the other algebra we missed along the way. So we’ll cover quadratics again, including all the factoring and solving stuff.

With that in mind, I thought I’d better make sure my students understand what a function is.

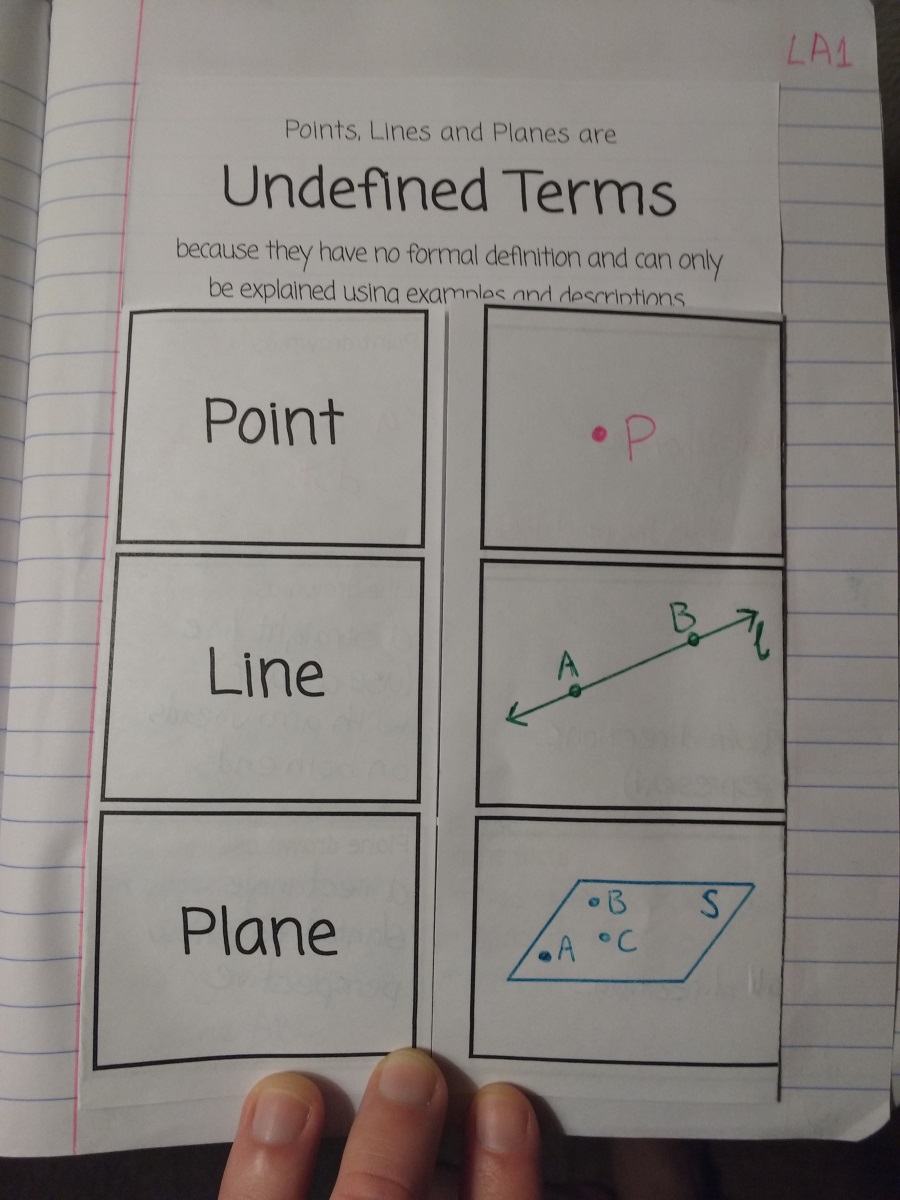
This is the front, basically just explaining what a function is. The examples on the bottom section were actually done after the notes inside. You could really use any examples you wanted. I chose these ones because this first unit focuses on absolute value functions, and I figured they should at least be able to evaluate quadratics, even if they don’t know anything else about them yet.

This is the part I’m most proud of. I think it lets students see clearly what the difference relation types are. I know it’s traditional to talk about the vertical and horizontal tests in this situation (and I did mention them briefly), but I decided to go back to the basic definitions of one-to-one, etc. and let students develop an understanding from those definitions. I was glad that by the time we did the last few diagrams, students were suggesting what the diagrams should be without any prompting.

Though they sometimes have to be prompted with “does each output have exactly one input,” most students have been good at recognizing the difference between one-to-one and many-to-one, which is critical as inverses are coming up soon. They often get many-to-one and one-to-many mixed up. They know which is which, they just get the names confused. I had a student say at one point, “So, it’s the number it goes from to the number it goes to, right?” Then after realizing what she’d just said, added “Oh, I guess that makes sense.” So, at least one of them has made that connection!

I know that it’s typical to make a mistake as a teacher and just claim it was to “see if you’re paying attention,” but in this case, having to cross out the bit that said “function” for one-to-many and many-to-many really was deliberate. (I promise I’m not just saying that!) Making students have to edit the page like this was an attempt to drive the point home that these are not functions. Thankfully, some of my students did notice that these sections contradicted the notes on the front of the foldable, and suggested it needed changing before I did.

Files are here.

This is the second skill for Algebra 2, which is pretty much what it says in the title.

At this point, we’re almost ready to start talking about functions, but I wanted to “review” a few things about solving equations first. Which brings us to these pages.

Except, lets just pause for a moment, and examine those quotation marks around “review”. The linear equation stuff is straight-up review, but solving absolute value equations is one of those used-to-be-in-2-but-now-in-1 topics I wrote about last time. Which means it’s new to my students this year, but should be something my future Algebra 2 students have already seen. With that out of the way…

Nothing terribly complicated here. These should be equations my students know how to solve, but as I suspected would be the case, they needed some help shaking some of the cobwebs loose first.

I find students get a lot more frightened of linear equations than they need to be, especially when fractions start showing up. This was a good chance to have them use a strategy that shows up again and again in algebra:

If you don’t know how to solve this problem, turn it into one you can solve.

And that’s the principle behind the design of these notes. Each situation shown really only requires one or two steps to turn it into an equation like the one above.

I would never suggest teaching this stuff for the first time like this. There needs to be much more time spent developing understanding at each stage. But for review, I think it worked fine.

I very purposely didn’t use “cross-multiplying” as the final strategy. Those words are banned in my classroom. While it could solve the example shown, cross-multiplying is useless for any problem involving more than two fractions, for instance. Every so often, I have a student suggest cross-multiplying as a way to solve a problem. Never have any of them been able to apply it the way they were supposed to. So, I’d rather my students take one more step to solve an equation and actually understand what is happening, rather than totally confuse themselves.

Absolute value equations are not in the Algebra 2 standards, but I wanted to include them anyway, because:

• These students missed them in Algebra 1. (Which means I’m supposed to cover them anyway.)
• AFAIK they’re on the ACT. They’d be some pretty easy points my kids would be throwing away if they didn’t know this.
• I’m about to use absolute value functions as an example for transformations and graphing, to set up everything we’re going to do with other functions. It’s probably a good idea if they knew how to find some x-intercepts, then.
• I think it’s going to be useful to expose students to equations that can have two, one or no solutions. I heard a rumor that there’re these things called quadratics that do something similar…
• I also wanted kids to practice finding extraneous solutions, because that’s going to come up soon, too.

Notice that the notes don’t point out that the absolute value function cannot equal a negative number. That was there originally, but I took it out, and let students solve a few equations first before we talked about it. I was hoping they’d realize themselves, but it did take a little bit of prompting for them to realize that something like |x – 2| = -3 has no solution without having to go through all the steps. In any case, it show the value of checking the solutions of an equation.

In case you were wondering, it is possible to get an absolute value equation that has one legitimate solution and one extraneous solution. Try something like |x – 2| = 2x.

Files are here

Our very first skill in Algebra 2 this year was this:

IN1: I can simplify radical expressions.

Even though it isn’t particularly related to the rest of the first unit (it’s called “Introductory Algebra Skills”, but it’s really functions and transformations, focusing on linear and absolute value functions), there are a number of reasons I wanted to start with this skill:

• In the change over to the new (now one year old) Oklahoma Academic Standards, this content migrated from Algebra 2 to Algebra 1. Meaning my students (who mostly took Algebra 1 two years ago) missed out. So, starting with this is one way of bridging the gaps between the two subjects and the two sets of standards.
• To a certain extent, this topic stands on its own, so I saw it as a way to get into our course quickly and let my kids start to get the feel of the rapid pace required in Algebra 2.
• The skill is not particularly difficult, assuming students have a strong grasp already of concepts like radicals, exponents and prime factors. This way, I get to quickly evaluate their understanding of these concepts, and hopefully rectify anywhere they’ve gone astray.
• Within the next few weeks, we’ll be graphing functions such as y = x² – 8. I would like for them to give me the x-intercepts as ±2√2.

I know that writing out all the factors individually isn’t the fastest method, but I’m a strong believer in the idea that we shouldn’t give students the fastest method first. In this case, there are a lot of shortcuts, but I’m consciously not teaching them explicitly. I’m certainly dropping a lot of hints that they exist. But if a student really understands the principles here, they’ll be able to find those shortcuts themselves. If a student isn’t able to find those shortcuts, that tells me they still need to develop their understanding of radicals until they can.

There are two key reasons why I think this method is solid, despite its slowness. Firstly, it always works for this type of problem, no matter how many factors the number has, or how many variables we throw under the radical. And secondly, it exposes what’s happening underneath all those strange mathematical symbols. Radicals remove repeated multiplications (or “exponents” as we like to call them). I want my students to see that repeated multiplication in their mind any time they see an exponent, even if they haven’t written it out.

I feel like this second page could be improved a bit. I state the “rules” for adding and subtracting radicals, but there’s not much in the way of justifying why these rules exist. Our class discussion did tease this out a bit more. But I think in future I want to put more emphasis about why addition and subtraction are possible to simplify in some situations but not others.

These are my thoughts right at this moment. We can simplify when we add and subtract between things we know the relative size of. For instance, we know 3x and 2x are respectively 3 and 2 times the size of x, regardless of whatever x happens to be. So we can add them and get 5x. But we can’t add 3x and 2y, because we don’t know how x and y relate to each other. (If we happen to actually know how they relate to each other, it turns out there is a way to add them.)

Same thing with √2 and √5, or ³√2. We don’t know how they relate. We could always express them as decimals, but that’s the thing we’re trying to avoid so we can maintain an exact value (and that’s trivial with a calculator anyway, and ∴ boring). 3√5 and 2√5 are related, on the other hand, so we can add them and get 5√5.

On the inside of each of these foldables is a blank grid. These are spaces for students to write practice questions in. For this sort of thing, I usually write a few questions on the board for them to fill in here. I like to make them up on the spot, because it lets me gauge how the group is going and adjust the examples I think they need to see. (I’ll admit, this does sometimes backfire as I’m standing at the board, and my mind goes blank.) I don’t typically give them enough questions to fill the whole page, but I encourage them to copy in any other practice questions they want to keep as examples, off of worksheets or wherever else. I like to remind them that their INB is their only reference they have access to on quizzes, so this is good motivation for some of them.

Files are here

This last week saw my Geometry classes finish off our introductory Reasoning and Logic unit, which means we’re ready to go with setting up the basic building blocks of geometry: the Undefined Terms.

I took similar notes last year, but they were constrained to a table on half a letter sheet. This year, I redesigned it to give us a bit more space.

My students questioned why we have terms that are undefined, especially after we went over the importance of good definitions in our last unit. I reminded them that definitions use existing terms to define new terms, but that can’t happen unless we have these few terms to build everything else on top of. (Is this a good explanation? Let me know if you know of a better reason to justify the undefined terms.)

Next was our first set of postulates and definitions for the year.

A change from last year is that these postulates are glued directly onto a notebook page, rather than folded inside a foldable. This is due to a decision I’ve made this year: all postulates, theorems and definitions will be visible immediately on the page they’re on. Examples, proofs and other content can be hidden in foldables. But if I’m going to expect students to look up certain parts of their notebooks frequently, I should make those parts easy to find.

Before we wrote these down, I did a little activity to get my students thinking about why these are true. I drew two points on the dry-erase board.

I didn’t get any photos of this, so I’m re-enacting these on my computer.

Then I asked if any volunteers could draw a line through them. That went well enough:

I asked if they could do this wherever I put the points. The consensus was that they could. So far, so good.

Then I asked if anyone could draw a different line that also went through the two points. Some kids started saying yes, but then took that back once they realized they didn’t know how to do it. One even got as far as standing at the board with a marker, before handing it back to me and saying it couldn’t be done. My plan had worked as I’d hoped. The class had figured out Postulate 1 without me having to tell them what it was.

After we had written Postulate 1 down, I set my next challenge. Draw two lines that intersect at one point. No-one wanted to stand up to do this, because they thought it might be another trick question. But eventually, I coaxed one student to the board, who drew something like this:

Then I asked for two lines that didn’t intersect at all. I heard a student say the word “parallel”, so I handed her the marker:

Then I asked if anyone could draw two lines that intersected twice. But they knew my game by this point, and let me know it couldn’t be done. So it was time to write Postulate 2.

We didn’t get through the rest of the notes in this lesson, but I’ve written them up in my notebook anyway.

If you want to download these, you can do so here:

• Undefined Terms
  .pub
  .pdf
• Points Lines and Planes: Postulates and Definitions
  .pub
  .pdf

Let’s cut to the chase. Here are my units with SBG skills (and alignment to the Oklahoma Academic Standards) for both Geometry and Algebra 2:

• geometry units.pdf
• algebra 2 units.pdf

Last year was my first year teaching under the American system, with brand new standards as well. There were some parts of my courses I was happy with, but a lot of things I’m changing this year.

I’m pretty happy with how Geometry went last year. I knew pretty much from the moment I was hired that I was going to be teaching it, so I had a lot of time to think about how I was going to arrange things.

The biggest change this year is moving Trigonometry from the end of the year. I realized as I went through other units that it would have been useful to have kids knowing how to use right-triangle trig to solve problems, especially ones involving area. I’ve also scrapped the introductory unit, which was mostly some Algebra 1 review and the Pythagorean Theorem. Pythagoras is joining the trig unit now, and I’ll review things like solving linear equations as the need arises.

Algebra 2 is changing a lot. Last year, my first non-introductory unit was Quadratics. I found the classes getting bogged down, not making a huge amount of progress. Having to cover both graphing as well as factoring and all the algebraic manipulation that goes along with it got to be a bit too much for my kids. I never really felt like we’d built a solid foundational context for the rest of the course to rest on.

This year, the start of the course is going to focus on functions, transformations and inverses. For quadratics that means only dealing with vertex form, as well as showing the relationship to square roots. We’ll cover all the functions that way, before coming back to all the other algebra we missed along the way. So we’ll cover quadratics again, including all the factoring and solving stuff.

While it’s not proper spiraling, I guess it’s sort of a slow spiral.

I’m also teaching Statistics this year, but I won’t be using SBG for it. I feel like I need to learn a bit more about how a standalone stats class works before I attempt that.